Chapter 6 Reversible Systems
Real systems are characterized by friction, turbulence, unrestrained expansion, temperature gradients and mixing of dissimilar substances and are therefore irreversible. Molecular disorder increases and the total entropy is no longer constant but is constantly increasing.
Reversible systems are “ideal" in the sense that no energy is”wasted". In order to understand reversibility better, we will now consider some reversible and irreversible processes.
6.1 Reversibility and Irreversibility
Consider a simple pendulum as shown in Figure 6.1 below. When the bobbin is released at an elevated position, the bobbin accelerates in the gravitational field and potential energy is converted into kinetic energy - as well as dissipated by friction at the pivot point, friction between the air and the pendulum and also because of turbulence in the air caused by the movement of the bobbin through the air.
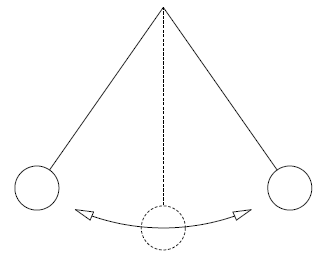
Figure 6.1: A simple pendulum.
At its lowest end the bobbin’s kinetic energy is at its maximum and its potential energy at its minimum. Then the bobbin decelerates and kinetic energy is converted into potential energy again until the bobbin reaches its highest elevation at the opposite end. The bobbin will swing back and forth until all its energy has been dissipated by friction and turbulence and the bobbin comes to a standstill at the lowest elevation. In order to keep the pendulum swinging, the environment has to do work on the pendulum to overcome the effect of friction and turbulence - like in a grandfather clock where the energy is supplied by a spring or weights. A frictionless pendulum in an evacuated space can in principle keep on swinging forever. In such a (reversible) pendulum, conversion between kinetic and potential energy takes place without any losses.
The two factors that make a pendulum irreversible are friction and turbulence which converts the kinetic and potential energy into heat which cannot be - even in theory, be fully converted back into potential or kinetic energy again. In the absence of these two factors the pendulum would have been reversible. Unrestrained expansion is another factor that makes a process irreversible. Consider a balloon that bursts. In order to restore the air that was inside the balloon to its original condition, work is required (to compress the air) as well as heat exchange with the environment (to lower the temperature after compression). The fact that outside action is required to restore the system to its original condition means the process is irreversible. It is possible (in principle) to expand air in a reversible manner. Consider an adiabatic frictionless piston cylinder arrangement with compressed air inside. Initially the piston is restrained by sand on top of the cylinder as shown in Figure 1.2 below:
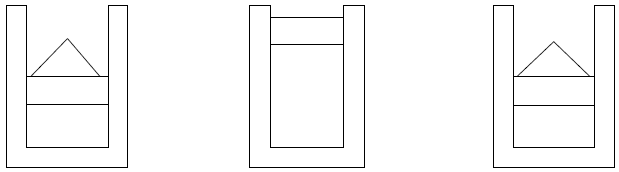
Figure 6.2: Reversible adiabatic expansion of a gas.
Grains of sand are removed one by one, allowing the gas to expand slowly enough to ensure that the pressure and temperature of the air inside stays uniform – in other words a quasi-equilibrium process. The process can now be reversed by placing the grains of sand back on the piston, compressing the gas slowly until the original state has been reached.41
Consider energy conversion during this expansion process. In the expansion of the gas, work is performed by the air molecules colliding with the piston, moving it upward. Some of the kinetic energy of the colliding molecule is therefore converted into work and the kinetic energy of the molecule is reduced. The total kinetic energy of the air molecules therefore drops. The internal energy (and the temperature) of the gas will therefore also decrease. During the compression of the gas work is performed and the temperature will rise again (because the velocity of the molecules are increased when they collide with the approaching boundary) to its original value.
A balloon that bursts in a vacuum (unrestrained expansion) is a irreversible process. The air does not perform work - and assuming an adiabatic process, the internal energy of the gas remains constant. Assuming air to be an ideal gas, the temperature of the gas will also remain constant.42 To compress the gas again, work must be performed on the gas and its temperature will rise. To return the gas to its original temperature, heat must be removed from the gas.
Heat transfer across a temperature difference is another factor that makes processes irreversible. Consider a piston cylinder arrangement containing \(1kg\) of saturated liquid water at \(100 ^\circ C\). In order for heat to be conducted across the walls of the container at a finite rate, heat at a slightly higher temperature than \(100 ^\circ C\) must be available. Let us assume a Thermal Energy Reservoir (TER)43 at \(110 ^\circ C\) supplies the heat and the water boils at constant pressure until a saturated vapour results as shown in Figure 6.3 below.
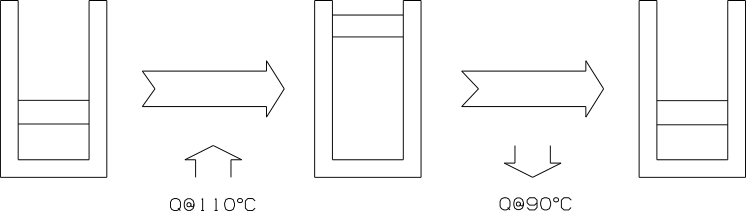
Figure 6.3: Irreversible heat transfer.
In order to reverse the process a TER at a lower temperature than \(100 ^\circ C\) must be available to act as a heat sink. Let’s assume heat is rejected to a TER at \(90 ^\circ C\). The vapour condenses at constant pressure and a saturated liquid at \(100 ^\circ C\) results again - so the water inside the piston cylinder is restored to its original condition, but the environment has changed. The temperature of the \(2258kJ\) of heat necessary to vaporize the water, has changed from \(110 ^\circ C\) to \(90 ^\circ C\). In a reversible process there will be no temperature gradient and the heat will be absorbed and rejected at the same temperature (\(100^{\circ}C\)) making it possible to reverse the process without changing the temperature of the heat.
Although not relevant in processes considered here, mixing of two dissimilar substances is also irreversible. Once the coffee granules have dissolved in the water, they cannot be restored to their original condition without considerable effort. We have identified four factors that make processes irreversible:
Friction and turbulence
Unrestrained expansion
Heat transfer over a finite temperature gradient
Mixing of dissimilar substances
In order to quantify the degree of (ir)reversibility, a new property, entropy is defined.
6.2 Entropy
From a statistical viewpoint, entropy is described as a measure of molecular disorder. Molecular disorder can be described in terms of position and in terms of velocity. For instance, particles at rest can be ordered or disordered. Also, particles moving at different velocities and directions are more disordered than particles all moving at the same velocity and direction. In the gas phase, the molecules move about at random, collide and change direction. Therefore the entropy of a substance should be higher in the gas phase and lower in the solid or liquid phase. Also, according to the kinetic gas model, the higher the internal energy of a gas, the higher the kinetic energy of the gas molecules. Therefore entropy should rise as heat is added to a gas, when a solid melts or a liquid boils to form a vapour. This leads to the first very important statement44 about entropy:
Entropy transfer accompanies heat transfer. In a reversible system this enables us to calculate the change in entropy using the definition of entropy:
\[\begin{equation} dS=\left(\frac{\delta Q}{T}\right)_{reversible} \tag{6.1} \end{equation}\]
where \(\delta Q\) is the differential amount of heat exchanged in a reversible process and T the temperature of the system boundary. In a reversible system there will be no temperature gradients in the system.
To illustrate how the flow of heat leads to a change in entropy, consider \(1kg\) of saturated liquid water at 100kPa in a closed system. The water is heated at constant pressure (and therefore also at constant temperature) until it is a saturated vapour. The heat necessary to vaporize the water is \(2\thinspace258kJ\) as calculated from the First Law. Because the temperature remains constant during the process, the equation above is easily integrated to give the change in entropy for the water upon evaporation:
\[\Delta S =\frac{Q_{in}}{T}=\frac{2258}{99.63+273.15}=6.057\frac{kJ}{K}\]
If the water is condensed again and the heat released at the equilibrium temperature, the system is back at its original condition and the heat is still at the same temperature it has been supplied to the system. In this case the evaporation and condensation were done infinitely slowly in order to make sure that the vapour and liquid were at the same temperature and there were no temperature gradients.
Lets consider a process where the temperature changes. A perfect gas is heated at constant pressure from \(T_1\) to \(T_2\) The heat required is given by:
\[\delta Q = C_pdT\]
replacing \(\delta Q\) in the definition of entropy and integrating gives:
\[\begin{equation} \Delta s = C_p \int_{T_1}^{T_2}\frac{dT}{T}=C_p\ell n \frac{T_1}{T_2} \tag{6.2} \end{equation}\]
It is possible, working from a suitable reference point, to calculate the value of entropy at any state. It can be shown that the value of entropy so calculated is independent of the calculation path and depends only on the reference state and the end state. This is a necessary and sufficient condition to make the following statement:
Entropy is a property Entropy can therefore be used in the specification of the state of a substance. For a single phase substance, if the value of two independent properties are fixed, the state is fixed. Entropy cannot be measured but can only be calculated. It is therefore an abstract property - like the momentum of a particle.
6.3 Determination of Entropy
We distinguish between three types of substance: Real and condensible substances, Ideal gases and Perfect gases (Ideal gases with a specific heat independent of temperature). As with other properties a distinction is made between specific entropy - the entropy per kilogram of substance, using the lower-case \(s\) with units of \([kJ/kg\cdot K]\) and total entropy using upper-case \(S\) with units of \([kJ/K]\).
6.3.1 Ideal gases
An equation giving the change in the entropy of an ideal gas can be derived using the differential form of the First Law for a closed system. Consider a unit mass:
\[\delta q = du + \delta w\]
For a reversible process \(\delta w = Pdv\) and for an ideal gas, \(P=\frac{RT}{v}\) and \(du = C_v dT\). Using these equations and the definition of entropy to replace the appropriate terms in the First Law, the following equation results:
\[Tds=C_vdT+RT\frac{dv}{v}\]
dividing by \(T\) and integrating gives:
\[\begin{equation} s_2-s_1=\int_{T_1}^{T_2} C_v\thinspace \frac{dT }{T}+R\thinspace \ell n \frac{v_1}{v_2} \tag{6.3} \end{equation}\]
Using the Ideal Gas Law \(v=RT/P\) to get rid of the volume terms in the equation above and remembering that \(C_p=C_v+R\) and rearranging, the following equation results:
\[\begin{equation} s_2-s_1=\int_{T_1}^{T_2} C_{p}\thinspace \frac{dT }{T}- R \thinspace \ell n\frac{P_2}{P_1} \tag{6.4} \end{equation}\]
Equation (6.4) is similar to Equation (6.2) but with a term to account for change in pressure. In general the specific heat \(C_p\) is not a simple function of temperature and in order to prevent having to do the integration every time a value for entropy is required, the entropy of different ideal gases as function of temperature, at \(100kPa\), relative to some reference point has been calculated using Equation (6.4) and tabulated as \(s^o_T\). To find the entropy at the same temperature T, but at a pressure (P) other than \(100kPa\), Equation (6.4) is used. Because the temperature stays constant, only the pressure term remains:
\[\begin{equation} s_{T,P}=s^o_T-R \thinspace \ell n\frac{P}{100} \tag{6.5} \end{equation}\]
Where \(s_{T,P}\) is the entropy at the specified temperature and pressure and \(s^o_T\) is the entropy at the specified temperature but at \(100kPa\). Its value can be found in tables giving the properties of gases under the assumption of ideal gas behaviour.
Example
Calculate the entropy of air at \(200kPa\) and \(25^\circ C\)
Solution
The value of entropy for air at \(25^\circ C\) and \(100kPa\),
\({s^o_{298.15K}}\) is read from the Ideal Gas Air tables. From Equation
(6.5):
\[s_{200kPa;25^\circ C}=s^o_{298.15K}-R_{air} \thinspace \ell n\frac{P}{100}\]
\[=6.863-0.287\ell n\frac{200}{100}=6.664kJ/kg \cdot K\]
The answer seems logical as one can expect the molecular disorder to decrease as the pressure increases.
Because entropy is a property it can be used to define the state of the air. Because air is a single phase substance the value of two independent properties must be specified to fix the state. For specified values for entropy and temperature, the pressure can be determined directly from Equation (6.5) but for specified values for entropy and pressure, an iterative procedure may be necessary to determine temperature from the tabulated values45. Once the values of temperature and pressure are known, the Ideal Gas Law can be used to calculate the specific volume.
6.3.2 Perfect gases
For the mono-atomic (ideal) gases (Ar, Ne, He, Kr, Zn) \(C_p\) is virtually independent of temperature and collectively they are called perfect gases. For a \(C_p\)46 independent of temperature Equation (6.4) is easily integrated to give the change of entropy with temperature and pressure:
\[s_2-s_1=C_{p}\thinspace \ell n \frac{T_2}{T_1}- R \thinspace \ell n\frac{P_2}{P_1}\]
The change of entropy with temperature and specific volume is obtained by integrating Equation (6.3)
\[s_2-s_1=C_{v}\thinspace \ell n \frac{T_2}{T_1} +R \thinspace \ell n\frac{v_2}{v_1}\]
In this case, because it is only possible to calculate the change in entropy, it is not possible to use entropy as one of the variables that define the state. The same is true for internal energy and enthalpy - because, for a perfect gas, only the change in \(u\) and \(h\) can be calculated. A combination of two other variables (\(P,T,v\)) must be used to fix the state.47
6.3.3 Compressed liquids
A change in pressure will not affect the entropy of a incompressible liquid. Assuming incompressibility for a compressed liquid (a good assumption at moderate pressures and a reasonable plan of action if compressed liquid tables are not available), the value of the entropy of a saturated liquid at the same temperature can therefore be used. Also, it is often also assumed that the specific heat of a compressed liquid does not change much with temperature. The change in entropy of a compressed liquid with temperature can then be calculated using Equation (6.2) as in the example on page .
6.3.4 Real and condensible gases
Values for entropy are tabulated in the steam tables for saturated water (as a function of pressure or temperature) and for super-heated water (as a function of pressure and temperature). Note also that the entropy of saturated liquid water at the triple point (0.01\(^\circ\)C and 0.6113kPa) is (arbitrarily) chosen as zero.
For a single phase substance, once the values of two independent properties are specified, the state is fixed while for a two phase mixture, temperature and pressure are no longer independent and only one of the two can be specified. For a specific set of variables, it is important to first determine the phase.
Example
Calculate the temperature and enthalpy of steam at \(10kPa\) with an
entropy of \(7kJ/kg\cdot K\)
Solution
The value for entropy is larger than the value for entropy for the
saturated liquid at this pressure (\(0.6493 kJ/kg\cdot K\)) but smaller
than the value for the saturated vapour (\(8.1502 kJ/kg \cdot K\)) This
means that it is a two phase liquid and the temperature is the
equilibrium value at \(10kPa\), \(45.81^ \circ C\). To determine the
enthalpy of the two phase mixture (TPM), the quality must be known. This
is calculated from the value of the entropy:
\[s_{TPM}=s_gx+s_f(1-x)\] \[7=8.1501x+0.6492(1-x)\] \[\therefore x=0.8467\] \[h_{TPM}=0.8467\times 2584.63+(1-0.8467)191.81=2217.7kJ/kg\]
According to the steam tables, the entropy of saturated liquid water at 100kPa is \(1.3026kJ/kg\cdot K\) and of saturated water vapour, \(7.3594kJ/kg\cdot K\). The change in entropy upon vaporisation is therefore \(6.0568kJ/kg\cdot K\).
6.4 Entropy and work
From the definition of entropy on page , it is clear that the entropy during an adiabatic reversible process will stay constant. During the adiabatic reversible expansion of a gas in a piston/cylinder set-up, work is performed but the entropy does not change. This means that work is "free" of entropy. This is also the case for open systems. As steam flows through an adiabatic reversible turbine, the entropy of the steam does not change (the entropy at the inlet and the outlet will be the same) although the turbine delivers power. From this follows the third very important statement about entropy:
There is no entropy transfer associated with the reversible energy transfer as work or power. This is in contrast to heat, where heat transfer leads to a change in entropy - as is evident from the definition of entropy in Equation (6.1).
The same argument is valid for the conversion of enthalpy into kinetic energy or potential energy. Consider a reversible adiabatic nozzle. The entropy at the inlet and outlet will be the same even though some of the enthalpy was converted into kinetic energy.48
6.5 Reversible processes
Because no heat transfer takes place, the entropy during an adiabatic reversible process does not change. Now consider heat exchange between a system and a heat source. For this process to be reversible, the system and the heat source must be at the same temperature. From the definition of entropy, it is clear that the increase in the entropy of the system will be the same as the decrease in the entropy of the heat source and this leads to the fourth very important statement about entropy:
For reversible processes, the total entropy (\(S_{total}=S_{system}+S_{environment}\)) remains constant49. This means that for a reversible process, we can do an entropy balance. This principle will now be demonstrated for the following processes:
Reversible adiabatic processes.
Reversible processes with heat transfer
Reversible heat engines and heat pumps - a special type of closed system.
6.5.1 Reversible adiabatic processes
Adiabatic reversible processes are known as isentropic processes.
6.5.1.1 Closed systems
Consider the reversible adiabatic compression of a gas
Because no heat flow has taken place, it follows from the definition of entropy, Equation (6.1), that \(S_2=S_1\)
Example
One kilogram of steam at \(200kPa\) and \(200^\circ C\) expands reversibly
and adiabatically to a pressure of \(50kPa\). Calculate the work.
Solution
As steam is not a perfect gas, there is no simple analytical
relationship between pressure and specific volume for an isentropic
process and we cannot use \(\delta W = P dV\) to determine work as was
done in Paragraph
3.2.3. However, the First Law contains a
work term and for this adiabatic process becomes: \[mu_1=mu_2+W_{out}\]
The internal energy at the end of the process (\(u_2\)) can be determined as a function of \(P_2\) and \(s_2\). The entropy will stay constant and we can calculate the value of entropy before the process has taken place. Therefore, \(s_2=s_1=7.5066\frac{kJ}{kg\cdot K}\). The steam is in the two-phase region with a quality of \(x_2=0.986\) and \(u_2=2455.1\frac{kJ}{kg}\).
\[W_{out}=m(u_1-u_2)=2654.4-2455.1=199.3kJ\]
6.5.1.2 Open systems
For an adiabatic, steady, open system it means that the rate at which entropy is entering the system with mass flow will be the same as the rate at which it is leaving the system with mass flow. For a system with only one inlet and one outlet:
\[\dot m s_i = \dot m s_u\]
Example
Helium \(600^\circ C\) and \(500kPa\) flows to a reversible adiabatic
turbine at a rate of \(2kg/s\). The pressure at the outlet is \(200kPa\).
Calculate the power.
Solution
An energy balance for the turbine gives:
\(\dot m h_{in}=\dot m h_{out}+\dot{ W}_{out}\). Rearranging:
\[\dot W_{out}=\dot m (h_{in}-h_{out})=\dot m C_p (T_{in}-T_{out})\]
The entropy will not change and Helium is a perfect gas, therefore:
\[s_{out}-s_{in}=C_{p}\thinspace \ell n \frac{T_{out}}{T_{in}}- R \thinspace \ell n\frac{P_{out}}{P_{in}}=0\]
The only unknown in this equation is \(T_{out}\) and its value is calculated as \(605.2K\), with the result that \(\dot W_{out}=2782.5kW\). As this is a perfect gas undergoing an isentropic process, it is also possible to use Equation (4.4) to calculate \(T_{out}\).
Example
Air at \(300K\) and \(295.7kPa\) is accelerated in a reversible ("ideal")
adiabatic nozzle. The pressure at the outlet is \(100kPa\). Calculate the
velocity at the outlet. Assume the inlet velocity is negligibly small.
Solution
An energy balance gives:
\[\dot{m} h_{in}=\dot{m}\Bigg ( h_{out,isentropic}+\frac{\overline V^2_{out,isentropic}}{2000} \Bigg)\]
Using Equation (6.5), the entropy at the inlet can be calculated:
\(6.5518kJ/kg.K\). The entropy at the outlet will be the same as the
entropy at the inlet. The enthalpy of air at \(100kPa\) with an entropy of
\(6.5518kJ/kg.K\) can be read directly from the ideal air table as
\(220.218 \thinspace kJ/kg\) and used in the energy balance to determine
the outlet velocity: \(400.6\thinspace \frac {m}{s}\). The isentropic
outlet temperature can also be read from the ideal air gas table:
\(220K\).
The nozzle in the example on page is a real nozzle. Although the outlet pressure is the same, the (real) outlet temperature is higher (\(240K\))because the conversion of the energy in the inlet air to kinetic energy is not as efficient and therefore the temperature does not decrease as much as in the reversible nozzle. Therefore the (real) outlet velocity is lower (\(353 \thinspace \frac{m}{s}\)). It is left to the reader to confirm that the entropy at the outlet of the real nozzle on page is higher than at the inlet.
6.5.2 Reversible processes with heat transfer
Heat is usually supplied by a heat source or rejected to a heat sink (often the environment). If we assume a uniform temperature inside the source or sink as well as an infinite heat capacity so that its temperature does not change, we call it a Thermal Energy Reservoir (TER). For a TER we also assume a constant volume (so no work is performed). The change in entropy of a TER can be calculated using the definition of entropy.
Example
Assume that the environment is a heat sink at \(25^\circ C\). Calculate
the change in the total entropy of the environment with the addition of
\(20MJ\) of heat.
Solution
From the definition of entropy:
\[dS=\left( \frac {\delta Q}{T}\right)_{reversible}\]
\[\Delta S_{environment}=\frac{20\thinspace 000}{25+273.15}=67kJ/K\]
6.5.2.1 Closed systems
When the system release (absorbs) heat its entropy will decrease (increase) and the entropy of the TER receiving (supplying) the heat will increase (decrease) by the same amount because the total entropy must stay constant.
Example
One kilogram of steam at \(200kPa\) and \(400^\circ C\) is expanded at
constant temperature to \(100kPa\) in a piston cylinder arrangement
exchanging heat with a TER at the same temperature. Determine the work
analytically.
Solution
The equation \(\delta W = P dV\) can be integrated numerically but that
would not be an exact solution. So another strategy must be devised. The
First Law for closed systems: \[Q_{in}=m(u_2-u_1)+ W_{out}\] The initial
and final temperatures and pressures are known, so the \(u_1\) and \(u_2\)
can be read from the steam tables. The amount of heat exchanged must
first be calculated from an entropy balance. The change in the entropy
of the steam is equal to the change in the entropy of the TER supplying
the heat: \[m(s_2-s_1)=\frac{Q_{TER,out}}{T_{TER}}\] Entropy can be read
from the steam tables and \(Q_{TER}\) calculated:
\[Q_{TER,out}=(8.5434-8.2217)\cdot(400+273.15)=216.55kJ\] \(Q_{TER,out}\)
is equal to \(Q_{steam,in}\). The First Law therefore gives:
\[W_{out}=216.55-(2967.85-2966.69)=215.39kJ\] of work done by the system
on the environment.
6.5.2.2 Open systems
For an open system it means that the rate of change of the entropy of the TER must be equal to the rate of change of the entropy of the substance. For a process where heat is supplied by the TER:
\[\frac{\dot Q_{TER,out}}{T_{TER}}=\dot{m}s_{out} - \dot{m}s_{in}\]
If the entropy associated with the heat, is seen as a "stream of entropy", an equivalent entropy conservation equation (rate of entropy inflow = rate of entropy outflow) can be formulated:
\[\dot{m}s_{in} + \frac{\dot Q_{TER,out}}{T_{TER}}=\dot{m}s_{out}\]
Example
Air at 300K and 100kPa is compressed at constant temperature to 200kPa
in an reversible compressor at a rate of \(2kg/s\) exchanging heat with a
TER at the same temperature. Determine the power required.
Solution
During compression, the kinetic energy of the gas molecules will
increase and the temperature of the air will rise if this energy is not
released as heat. An enthalpy balance gives:
\[\dot m h_{in} + \dot W_{in}=\dot m h_{out}+\dot Q_{out}\] Assuming air
to be an ideal gas, enthalpy is not dependent on pressure and
\(h_{in}=h_{out}\). This means that the power required is the same as the
heat transfer rate. In order to calculate the heat transfer rate an
entropy balance is performed. Because this is a reversible process, the
total entropy stays constant and the rate at which the entropy of the
air decreases must be the same as the rate at which the entropy of the
TER increases:
\[\dot m (s_{in}-s_{out})=\frac{\dot {Q}_{TER,in}}{T_{TER}}\] For air:
\[s_{in}-s_{out}=s^o_{300K}-(s^o_{300K}-R_{air} \thinspace \ell n \thinspace\frac{200}{100})\]
and the heat transfer rate becomes:
\[\dot Q=300\times 2\times 0.287\ell n \thinspace 2=119kW\] and:
\[\dot {W}_{in} = \dot Q = 119kW\]
6.5.2.3 Unsteady state processes
Entropy can also be used to solve reversible unsteady state problems. Consider a system where heat is exchanged.
Example
Air at \(25^\circ C\) and 100kPa flows to a isothermal reversible
compressor. The compressor releases heat at a sufficient rate to the
environment (also at \(25^\circ C\)) to ensure that the air at the outlet
of the compressor is at \(25^\circ C\). The air from the compressor flows
to a rigid tank of \(2m^3\). The tank is initially filled with air at
\(100kPa\) and \(25^\circ C\). The tank releases heat at a sufficient rate
to the environment to ensure that the temperature of the air in the tank
also remains on \(25^\circ C\). The process continues until the pressure
in the tank reaches 1000kPa. Calculate the power required by the
compressor. All processes are reversible.
Solution
An entropy and energy balance over the compressor alone cannot be used
to solve the problem as the value of entropy of the air at the outlet of
the compressor is not constant, it changes as the outlet pressure of the
compressor rises.
Consider the whole system. (It is left to the reader to draw a picture.) An energy balance gives: \[m_1u_1+m_i h_i+W_{in}=m_2 u_2 + Q_{tot}\] Where \(Q_{tot}\) is the heat released by the compressor and the tank. We can determine \(m_1\) and \(m_2\) from the Ideal Gas Law and \(m_i\) from a mass balance. We can read the values for enthalpy and internal energy from the tables, but it still leaves two unknowns, the work and heat transfer. However, as the process is reversible, the total entropy remains constant and the entropy at the beginning plus entropy in = entropy at the end plus entropy out: \[m_1s_1+m_i s_i=m_2 s_2 + \frac{Q_{tot}}{T_{air}}\] The total amount of heat released can now be calculated: \[Q_{tot}=298.15(2.337\times6.863+21.04\times6.863-23.37\times6.202)=4606 kJ\] The energy balance can now be used to calculate the work required by the compressor: \(2805kJ\).
The example above has been carefully formulated to make a direct solution possible. For instance the temperature at which heat is exchanged remained constant, making it possible to formulate a single equation for the entropy balance. This is not always possible. An incremental approach may then solve the problem. Consider the following example.
Compressed air storage is sometimes proposed as a way to store energy. Consider a pressure vessel filled with compressed air. The air is used to power an isentropic turbine. Assume that air is a perfect gas. The volume of the rigid, adiabatic pressure vessel is known. For an perfect gas, the values of the specific heats are constant and the value of the gas constant is known. The following equations can be formulated and solved from an initial (\(i=0\)) to a final condition (\(i=N\)) using a program like EES or a spreadsheet. Typically the values of the initial temperature and pressure will be known and the vessel will be discharged to a predetermined pressure. The number of increments (\(N\)) is made sufficiently large to ensure that \(\delta m = \frac{m_1-m_2}{N}\) is sufficiently small that the property values do not change too much during each increment. (The value of \(m_2\) cannot be determined at this stage as the value of \(T_2\) is still unknown. Some trail and error may be therefore be necessary to find a suitable value for \(N\). The values of \(T_{ref}\) and \(P_{ref}\) can be set equal to the initial conditions or another suitable value.)
The equations are:
Mass balance: \[\begin{aligned} m_i&=m_{i+1}+\delta m\\ m_i&=\frac{P_i V}{R\thinspace T_i}\\ m_{i+1}&=\frac{P_{i+1} V}{R\thinspace T_{i+1}} \end{aligned}\]
Energy balance: \[\begin{aligned} m_iu_i&=m_{i+1}u_{i+1}+h_{out}\delta m + \delta W_{out}\\ u_i&=C_v(T_i-T_{ref})\\ u_{i+1}&=C_v(T_{i+1}-T_{ref})\\ h_{out}&= C_p((T_i+T_{i+1})/2-T_{ref}) \end{aligned}\]
Entropy balance:
\[\begin{aligned} m_is_i&=m_{i+1}s_{i+1}+s_{out}\delta m\\ s_i&=C_p\thinspace\ell n\frac{T_i}{T_{ref}}-R\thinspace\ell n\frac{P_i}{P_{ref}}\\ s_{i+1}&=C_p\thinspace\ell n\frac{T_{i+1}}{T_{ref}}-R\thinspace\ell n\frac{P_{i+1}}{P_{ref}}\\ s_{out}&= (s_i+s_{i+1})/2 \end{aligned}\]
It is left to the reader to show that the heat loss during compressed air storage dramatically lowers the amount of work delivered by the turbine when discharging the compressed air through the turbine. When heat is lost during storage, the internal energy decreases, reducing the value of the enthalpy of the compressed air flowing to the turbine. In order to begin to effectively store energy using compressed air, the heat loss during storage must be minimized. This may be expensive.
Unfortunately, the process of charging an adiabatic tank with air using an isentropic compressor is an irreversible process. This is because the temperature of the air from the compressor rises as the air pressure in the tank increases with the result that air flowing into the tank is at a different temperature than the air already in the tank resulting in an irreversible mixing process and an entropy balance cannot be formulated. As one less equation is available, the value of one additional variable need to be specified. It may be necessary to use a differential approach again to calculate the work.
Using the property functions of EES the mass, energy and entropy balances can be solved for ideal or real gases.
6.5.3 Reversible heat engines
A heat engine is used to convert heat into (shaft) work. Heat engines form the basis of electricity generation where they are used to convert the heat released by the burning of coal or fissile nuclear material or solar energy to shaft work to turn an electric generator and also in auto-mobile engines where the heat released by the combustion of gasoline is converted to the work necessary to turn the wheels of the car.
Consider a reversible heat engine (RHE) based on a Carnot cycle. Saturated water at high pressure and temperature enters a boiler and leaves as saturated vapour at the same pressure. The saturated vapor enters an adiabatic reversible turbine and shaft work is produced. The two-phase mixture that leaves the turbine is almost completely condensed at constant pressure and temperature and enters an adiabatic reversible pump with an outlet pressure equal to the inlet pressure of the boiler. This saturated liquid enters the boiler to complete the cycle. The components and flow direction of the Carnot heat engine is shown in Figure 6.4 below. The high temperature TER supplies heat at the evaporating temperature and steam condenses at the same temperature as that of the low temperature TER.

Figure 6.4: Carnot heat engine.
A coal-fired power plant (and most nuclear plants) contains a somewhat similar heat engine. The burning of coal (or fission of uranium atoms) supplies the high temperature heat and low temperature heat is rejected to the environment. The power produced by the RHE is the power generated by the turbine minus a small percentage necessary to drive the pump. If we take the reversible heat engine as a black box with high temperature heat entering and power and low temperature heat leaving as shown in Figure 6.5, we can calculate the maximum (reversible) rate that power can be produced from high temperature heat. First we do an entropy balance.

Figure 6.5: Black box heat engine.
Because all the processes involved are reversible the total entropy will be constant. Therefore the rate at which the entropy of the high temperature TER decreases, will be the same as the rate at which the entropy of the low temperature TER increases. No mass flow is taking place across the boundaries of the heat engine. Therefore:
\[\begin{equation} \frac{\dot Q_H}{T_H}=\frac{\dot Q_L}{T_L} \tag{6.6} \end{equation}\]
The first law states that the energy must be conserved. Therefore:
\[\dot Q_H=\dot W_{out}+ \dot Q_L\]
Example
A reversible heat engine receives heat at a rate of \(10kW\) from a high
temperature reservoir at \(1000K\) and rejects heat to the environment at
\(25^\circ C\). Calculate the power delivered.
Solution
From Equation (6.6) the heat rejected to the environment can be
calculated: \[\frac{\dot Q_{amb}}{25+273.15}=\frac{10}{1000}\]
\[\dot Q_{amb}=2.98kW\] An energy balance gives:
\[\dot W = \dot Q_H - \dot Q_{amb}=7.02kW\]
This is quite high. In actual fact, a coal power station typically converts a third of the energy in the coal into electricity.
The heat engine can be reversed to give a heat pump - a device that “pumps” heat against the temperature gradient from a low temperature to a higher temperature. In a heat pump, the turbine is reversed, becoming a compressor (requiring energy) and the pump becomes a liquid-driven turbine.50 Heat pumps find application in refrigerators where heat is pumped from the inside of the refrigerator to the environment, air conditioning as well as for water heating where heat is extracted from the environment and used to heat water for domestic use.
Example
A typical domestic hot water cylinder contains \(200kg\) of water. Compare
the energy necessary to raise the temperature from \(15^\circ C\) \((T_1)\)
to \(60^\circ C\) \((T_2)\) using an electrical resistance heater with the
energy required by a reversible heat pump extracting heat from the
environment at \(15 ^\circ C\) \((T_{amb})\).
Solution
Assuming a constant specific heat of \(4.184 kJ/kg\cdot K\) for water,
Equation (5.7)
can be used to calculate the heat necessary to heat the water:
\[Q_{water}=mC_p(T_2-T_1)=200\times4.184(60-15)=37\thinspace 656kJ\]
This heat has to be supplied by the electrical resistance heater and is therefore equal to the electrical energy required.
To calculate the work required by a reversible heat pump, we first do an entropy balance. The entropy of the water will increase by the same amount as the decrease in the entropy of the environment because for a reversible system, the total entropy stays constant. Because the temperature of the water changes, Equation (6.6) cannot be used. Assuming water to be incompressible and \(C_p\) to be independent of temperature Equation (6.2) can be used to calculate the change in entropy of the water:
\[\begin{aligned} \Delta S_{water} & = m \thinspace(s_2-s_1) & & \\ & = m \int_{T_1}^{T_2} \frac{C_p dT}{T} & &\\ &= 200 \times 4.184 \times \ell n\thinspace \frac{60+273.15}{15+273.15} & = 121.4 \frac{kJ}{K} &= \frac{Q_{amb}}{T_{amb}}\\ \end{aligned}\]
(The change in entropy can more accurately be determined from the tables for compressed liquid water, or assuming liquid water to be incompressible, using the entropy of saturated liquid water at the appropriate temperature. It is left to the reader to confirm that the final answer is not significantly affected by using any of these methods.)
\[\therefore Q_{amb} = T_{amb}\thinspace \Delta S_{water}= 288.15\times 121.4 = 34990kJ\] From an energy balance around the heat pump (the amount of heat required to raise the temperature of the water remains the same): \[W_{RHP,in} =Q_{water}-Q_{amb} = 2666 kJ\] This work is supplied by the electric motor driving the compressor.
If energy is consumed/produced at a rate of \(1kW\) for one hour (\(3600 sec\)) the amount of energy is \(1kWh\) which is equal to \(\frac{1kJ}{s}\times 3600 s = 3600kJ\). Municipalities measure domestic electricity consumption in units of \(kWh\). Assuming electricity costs \(\frac {R1}{kWh}\), the cost to heat the water using a resistance heater is R10.46. Assuming a hot water consumption of \(200\ell/day\) results in an annual cost of \(R3818/year\).51
The energy required by the resistance heater as well as the energy required by the electric motor driving the compressor of the heat pump will be supplied by electricity. The (reversible) heat pump requires only \(7\%\) of the electricity required by the resistance heater. For actual heat pumps this figure is between 25 and \(30\%\). The running cost (electricity) of the heat pump will be lower but additional capital outlay will be necessary to purchase and install the heat pump. Assuming the heat pump reduce the consumption of electricity by \(75\%\), and that you want to pay back the purchase of the heat pump in five years, you can spend \(R3818\times0.75\times5=R14317\) on the installation of a heat pump.52
Another way of reducing electricity consumption is to install a solar water heater. A properly sized solar water heater can reduce the electricity consumption necessary to heat water using the geyser by \(80\%\) - increasing this percentage means a substantial increase in required hot water storage capacity (and cost) to provide for days with low or no sunshine. As a rule of thumb, heating water in a typical domestic household is responsible for 40% of the household’s total electricity consumption - in other words a solar water heater can reduce the household electricity consumption by \(40\%\times 0.8=32\%\).
Heat pumps and solar water heaters will be at their most economical where there is a constant consumption of hot water throughout the year - so the saving in running cost can cover the capital expenditure associated with the purchase of the heat pump or solar water heater.
A refrigerator also uses this principle to pump heat from the inside of the refrigerator (at a low temperature) to the environment at a higher temperature.
6.6 The Second Law of Thermodynamics
Thermodynamics has two fundamental laws: the First Law which deals with the conservation of energy and the Second Law, which describes the direction and efficiency of energy conversion. In most textbooks the Second Law is used as the starting point for the explanation of entropy.
A paraphrase of the Second Law as formulated by Clausius: “Heat will not spontaneously flow from a lower temperature to a higher temperature.”(Sonntag and Borgnakke 2012 Par 7.2)
This seems evident from our everyday experience where we find that a body at a higher temperature than its environment spontaneously loses heat but that in order to transfer heat from a lower temperature to a higher temperature require work – as for example in a refrigerator where heat from the inside of the refrigerator must be transported to the environment outside the refrigerator which is usually at a higher temperature than the inside of the refrigerator. A refrigerator requires energy in the form of electricity to power the electric motor which provides the mechanical energy (shaft power) to drive the compressor, which is a turbine in the heat engine mode (Paragraph 6.5.3).
Consider a refrigerator that violates the Second Law. In order to freeze water to make ice cubes, heat spontaneously flows from the inside of the refrigerator to the environment. Assume \(100kJ\) of heat at \(0^\circ C\) is transferred spontaneously (i.e. without requiring external work) to the environment at \(300K\). As no work is involved, \(100kJ\) of heat is released at \(300K\). The entropy of the water will decrease by \(\frac{100}{273}=0.366\frac{kJ}{K}\) while the entropy of the environment will increase by only \(0.333\frac{kJ}{K}\). We see therefore that a violation of the Second Law led to a decrease of the total entropy which is not possible as the total entropy can never decrease.
A paraphrase of the Second Law as formulated by Kelvin-Planck: "It is impossible to fully convert heat into work in a continuous manner." This means \(Q_L\) in Figure 6.5, can never be equal to zero. According to Kelvin-Planck there will always remain some low-temperature heat that will flow to the low temperature heat sink.
Consider a heat engine where the high temperature heat is fully converted into work. No low temperature heat remains that can flow to the low temperature reservoir. The entropy of the high temperature TER would decrease, while the entropy of the low temperature TER will remain constant. This means the total entropy would decrease which we know is not possible.
Perpetual motion machines can usually be shown to be impractical because they either violate the First Law by creating energy or the Second Law by reducing the total entropy.
6.7 Enthalpy revisited
Enthalpy is defined as the sum of internal energy (\(u\)) and what is often called “flow energy” (\(Pv\)) which is difficult to conceptualize. The application of the First Law on open, steady state, reversible processes, gives another view of enthalpy that may make the concept of “flow energy” easier to grasp. Consider water flowing in an adiabatic pipe. Also replace enthalpy (\(h\)) with \(u+Pv\) or rather \(u+\frac{P}{\rho}\):
\[u_i+\frac{P_i}{\rho_i}+\frac{{\bf\overline V}_i^2}{2000}+\frac{gZ_i}{1000} = u_e+ \frac{P_e}{\rho_e} +\frac{{\bf\overline V}_e^2}{2000}+\frac{gZ_e}{1000}\]
The four types of energy represented here are: \(u\): internal energy, \(\frac{P}{\rho}\): flow energy, \(\frac{1}{2}{\bf\overline V}^2\): kinetic energy and \(gZ_i\): potential energy. If there is friction or turbulence, some of the flow, kinetic or potential energy will be converted into internal energy. In a reversible system there is no friction and if the fluid is incompressible (an assumption often made for liquid water), the internal energy will not change and the First Law above reduces to:53
\[\frac{P_i}{\rho}+\frac{{\bf\overline V}_i^2}{2000}+\frac{gZ_i}{1000} = \frac{P_e}{\rho} +\frac{{\bf\overline V}_e^2}{2000}+\frac{gZ_e}{1000}\]
This is also known as the Bernoulli equation and its units are \([kJ/kg]\)
Example
A man uses a hose pipe to wash debris from the gutters of his house. The
exit of the hose pipe is \(3m\) higher than the inlet of the hose pipe at
the tap. Due to a nozzle at the end of the hose pipe the water is
accelerated from \(5m/s\) at the inlet to an unknown value at the outlet.
The municipal pressure of the water at the tap is \(300kPa\) and the
ambient pressure \(85kPa\). Assuming a constant density of \(998kg/m^3\) for
the water and reversible (frictionless) flow, calculate the velocity at
the outlet of the nozzle.
Solution
From the Bernoulli equation:
\[\frac{300}{998}+\frac{5^2}{2000}= \frac{85}{998}+\frac{{\bf\overline V}^2}{2000}+\frac{9.81\times 3}{1000}\]
Therefore: \[V_{out} = 19.92 m/s\]
With the increase in elevation, the potential energy will increase resulting in a decrease in flow energy (a drop in pressure). At the nozzle the water is accelerated, the pressure drops to ambient and the remaining flow energy is converted into kinetic energy.
Consider the other extreme: a thin adiabatic pipe with high friction (and to keep it simple, no change in kinetic and potential energy). Due to friction the temperature of the water will increase. All the flow energy is therefore converted into internal energy. The Bernoulli equation cannot be used as it is not frictionless flow and anyway the Bernoulli equation does not have a term which is a function of temperature. The First Law must therefore be used (internal energy is a function of temperature): \[\frac{P_{in}-P_{out}}{\rho}=\Delta u = C_v\Delta T\] \[\Delta T =\frac{(300-85)}{4.184\times 998}=0.052^\circ C\] This is small and with liquid flow in pipes, the change in temperature is usually ignored. The small change in temperature is due to the high density of the water. Pressure drop and the associated energy loss due to friction can be calculated independently using a friction factor.
The density of gases is dependent on pressure and in general, even for isentropic flow, the internal energy will not stay constant.54 Therefore the Bernoulli equation is invalid for gases. While it is true for incompressible fluids, the perception also exists with gases, that energy content is determined by pressure. When inflating a bicycle tire with a hand (or foot) pump the impression is created that our energy is only used to raise the pressure of the air. The fact that a pressure difference provides the driving force for flow and there is therefore always a pressure drop over a turbine, strengthens the perception that the energy content is because of its pressure. Consider a system where there is a significant work interaction between the system and the environment, in this case the isentropic Helium turbine in Paragraph 6.5.1.
Under the assumption of perfect gas behaviour, the internal energy changes by:
\[\Delta u=C_v(T_{in}-T_{out})=3.11\times(700-461.8)=742.23kJ/kg\]
while the flow energy (\(Pv=RT\)) changes by:
\[\Delta Pv=R(T_{in}-T_{out})=2.077\times(700-461.8)=494.74kJ/kg\]
The change in enthalpy gives the energy delivered by the turbine and is equal to the sum of these two figures: \(1237kJ/kg\). In this case internal energy makes a bigger contribution than flow energy - \(\frac{742}{1237}=60\%\).
For ideal gases, this percentage is determined by the ratio of \(\frac{R}{C_p}\). For air this ratio is only \(\frac{0.287}{1.004}=28.5\%\). An isentropic turbine running on air with the same inlet temperature and between the same pressure limits as the Helium turbine above, will deliver only \(191kJ/kg\) shaft work with only \(52kJ/kg\) supplied by flow energy. Unfortunately, while delivering more energy, compressing the Helium will also require more energy per kilogram.55
6.7.0.0.1 A note on assumptions.
The incompressibility of liquid water and Ideal and perfect gas behaviour are assumptions made to simplify calculations and better illustrate relationships between different variables. When in doubt whether an assumption is valid, make the assumption and do the calculations. Now, using the answers obtained, check whether the assumption is valid. For instance, when assuming perfect gas behaviour, check how much the specific heat has changed during the process; in the case of Ideal gas behaviour, determine how much the compressibility factor deviates from unity (see the footnote in Paragraph 2.2.2) or whether a change in pressure affected enthalpy.
Software packages such as EES, make it possible to do calculations without making these assumptions but this may rob you of the opportunity to apply and develop your engineering judgement and obtain answers without properly understanding the fundamentals.
It can be argued the effort from outside is necessary to remove and replace the grains of sand. If this experiment was actually performed, it would have been a valid argument. But conducting this as a thought experiment, no effort is required to remove the grains of sand, suspend them in the gravitational field and replace them again. Even in a thought experiment, it is necessary to perform work when compressing the gas from the balloon.↩︎
Joule performed an experiment in 1843 where he connected two tanks with a valve. The one tank was empty (vacuum) and the other contained pressurized air. He opened the valve and found that the temperature stayed constant, leading him to the conclusion that internal energy of air does not depend on pressure. He later showed that for gases that deviate significantly from ideal-gas behaviour, internal energy does depend on pressure also.↩︎
A TER is a body with an infinitely large heat capacity (and constant volume) and can therefore supply and receive heat without its temperature changing. Because its volume stays constant, the TER also does not perform any work.↩︎
In this chapter we will encounter a few of these statements about the nature of entropy.↩︎
Use the values in the example above to illustrate this for yourself.↩︎
Tables giving the properties of gases usually give the values at a specific temperature. Under the assumption of a constant specific heat this value can be used at any temperature.↩︎
Manipulation of the equations for calculating the change in entropy, for a perfect gas undergoing a reversible, adiabatic processes (\(s_2=s_1\)), will yield Equations (4.4).↩︎
Additional textbook reading. In his book "The Exergy Method of Thermal Plant Analysis" paragraph 2.1, TJ Kotas explains the concepts of ordered and disordered energy and their relationship to entropy.↩︎
For a more elaborate proof of this statement see Cengal (Cangel and Boles 2002, 307).↩︎
For the sake of mechanical simplicity, in real, practical heat pumps instead of the liquid-driven turbine a throttling valve or a capillary tube is used to limit the flow rate of the working fluid and maintain the pressure differential between the outlet of the condenser and the inlet of the evaporator.↩︎
The daily consumption of warm water in a typical suburban household is roughly \(50\ell/person\).↩︎
This calculation is valid if it is assumed that the the rate at which the cost of electricity will rise is the same as the interest rate on the money used to purchase the heat pump.↩︎
A more rigorous derivation can be found in “Thermodynamics” by S Klein and G Nellis (Klein and Nellis 2012, 404).↩︎
If in the example above, if ideal gas air flowed in the thin pipe instead of water, the temperature would also have remained constant as it is an isenthalpic system and the First Law reduces to \(h_{out}=h_{in}\), as there is no work or heat interaction with the environment and for an ideal gas, enthalpy is independent of pressure. Interestingly enough, the internal energy would also have stayed constant as for an ideal gas internal energy is also independent on pressure. Because of the change in pressure, the density drops with the result that the flow energy also remains constant. Due to the friction and resultant pressure drop, the entropy will increase. If the air was used to power a turbine, the temperature would have dropped.↩︎
The difference when expressed as \(kJ/kmol\) is much smaller.↩︎